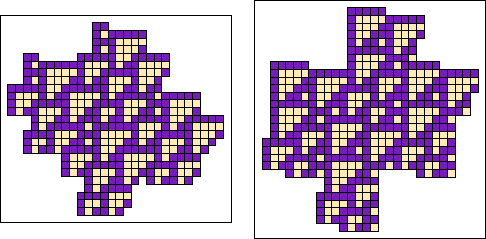
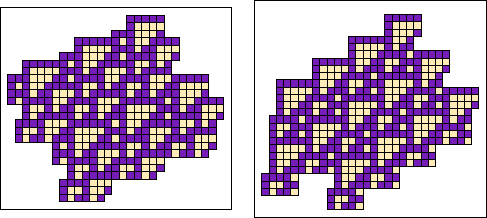
| |
10 |
9 |
8 |
7 |
6 |
5 |
4 |
3 |
2 |
1 |
0 |
1 |
2 |
3 |
4 |
5 |
6 |
7 |
8 |
9 |
10 |
| 1 |
. |
D2 |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
| 2 |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
37 |
. |
| 3 |
. |
D1 |
. |
74 |
. |
37 |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
| 4 |
. |
25 |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
| 5 |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
37 |
. |
. |
. |
. |
. |
. |
| 6 |
37 |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
| 7 |
17 |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
17 |
. |
. |
. |
| 8 |
. |
D2 |
. |
. |
. |
. |
. |
. |
. |
37 |
. |
. |
. |
. |
. |
. |
74 |
25 |
. |
. |
. |
| 9 |
161 |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
| 10 |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
. |
D |
. |
f |
. |
. |
. |
37 |
. |
. |
|