A. Irregular Leaves

Nowhere in the discussion of the flexagon of cycle greater than 3 has the angle between the various sides played a crucial position. It is true that we can create a relationship between the angles of the leaves and the angles of the map polygons, but this relationship is soon seen to be purely superficial. What is important in the map is not the shapes of the polygons involved, but the number of sides they have, i.e., as predicted, the cycle. It would seem therefore that, as in the triflexagons, we can change the angles and lengths of the sides of the leaf polygons, yet maintain the same mode of operation as in corresponding flexagons with regular leaves. Of course, more units may be required to allow flexing at all the faces, thus possibly decreasing the tendency of the flexagon to hold together at certain other faces (the same was true in the triflexagons). However, we will assume that an unlimited number of hands are available to operate the flexagon once it has been constructed, and go right ahead without considering practical difficulties. As in the triflexagons, we make the irregular polygons coincide in the assembled flexagon, and use at least $180^{\mbox{o}} a$ units, where $a$ is the smallest angle of the leaf.

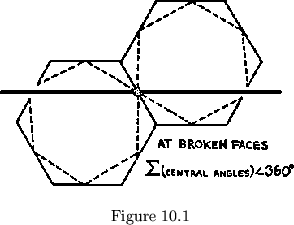
What happens if not enough units are employed? Those faces at which the angles pointed toward the center do not make up $360^{\mbox{o}}$ will not permit further flexing. The effect on the map is as if the face were broken in half (See fig. 10.1). At which faces will this occur? As in triflexagons, any two map paths, lying in adjacent cycles the midpoint of whose common side is in line with the midpoints of the two paths, represent faces having like angles at the center of the flexagon. This fact can help us in the manufacture of flexagons with irregular leaves. If we can indicate faces on the map at which like angles of the flexagon will be pointed toward its center, then each map path may be marked with the name of some such angle corresponding to an angle of the irregular leaf. Each face of the map must then be marked so that the angles of the hinge network polygons are identified with angles of the leaves, the angles occurring in the same order in the two places. One convenient way of doing this is by making the map so that the hinge network polygons will be similar to the leaf polygons. In building the plan, one leaf vertex between the incoming hinge of each leaf polygon and the next hinge (where we are traveling about the leaf in the direction, + or -, indicated by the sign corresponding to that leaf) must have an angle equal to the leaf angle designated for that leaf by the appropriate face in the map. Differently labeled angles in the plan should never have a common vertex.

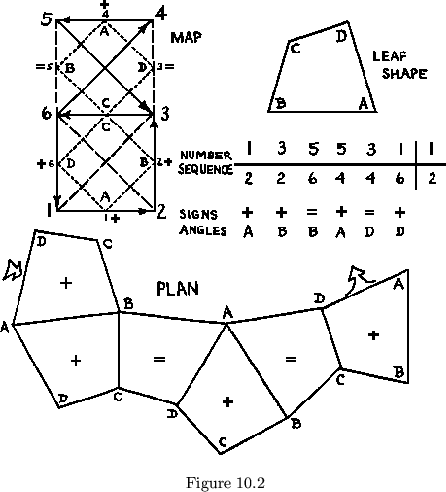
For example, to build the improper flexagon shown in fig. 10.2 with the irregular polygon $ABCD$ so that the angle $A$ would be at the center of the flexagon at face 1, we would proceed as shown.

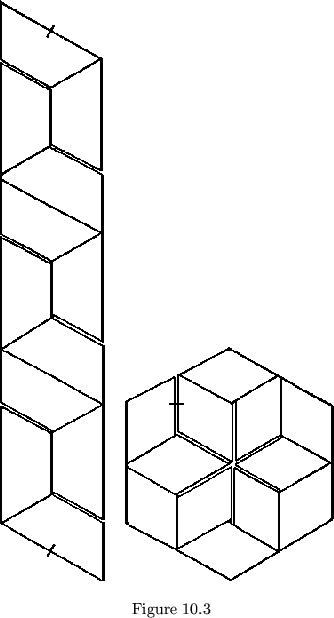
The proper tetraflexagon of order 4 has two interesting plans made from irregular leaves. The first is made from a hexagonal array of rhombuses and the second from a straight strip of isosceles trapezoids (see fig. 10.3). As is seen, the results of alternating leaf shapes are even more interesting in higher cycle flexagons than in triflexagons. They are so varied that other examples will be mentioned as needed, rather than all presented here.