next up previous contents
Next: Requirements Up: Playing Cards Previous: C. Pat Structure System.   Contents

DUALS

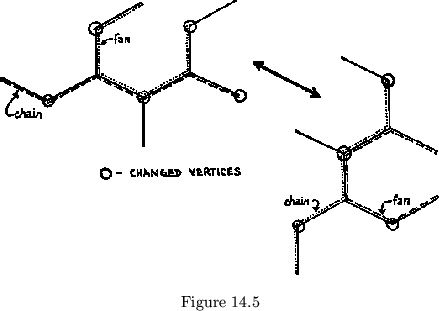
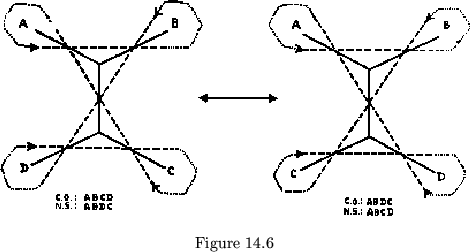
More generally, we might ask if, given any constant order, and thus the flexagon specified thereby, might there not be another, flexagon having this constant order as its number sequence? This does, in fact, turn out to be the case, and, moreover, the constant order of the second flexagon is found to be the number sequence of the first. That is, the two flexagons are duals. And, furthermore, the proof of this fact is constructive in nature; we describe a process for obtaining the Tuckerman tree of the dual directly from the original tree. Essentially, all the chain structures in the tree must be converted to fan structures, and vice versa. More precisely, alternate tree vertices must have the branches inverted in order, so that the righthand most branch becomes the lefthand most, etc. (See fig. 14.5). That this construction actually provides the dual is shown by induction from the segment of tree shown in figure 14.6, which has the dual shown there. All trees are made up of segments of this form, essentially, and dualizing then merely reverses the orientation of one vertex with respect to the next. The generalization of this process to flexagons of any cycle is clear.

\begin{figure}\centering\begin{picture}(290,175)(0,0) \put(0,0){\epsfxsize =290pt \epsffile{dibujos/fige05.eps}} \end{picture}\\ Figure 14.5 \end{figure}

\begin{figure}\centering\begin{picture}(320,170)(0,0) \put(0,0){\epsfxsize =320pt \epsffile{dibujos/fige06.eps}} \end{picture}\\ Figure 14.6 \end{figure}

Since every flexagon has a dual of the same order, but there are an odd number of flexagons of some orders, it follows that some flexagons are self-dual. Thus, among tri-flexagons of order 6, the fan and chain are dual, and the regular flexagon is self-dual (See fig. 14.7) 14.1.

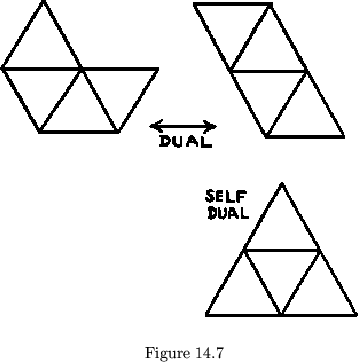
\begin{figure}\centering\begin{picture}(250,210)(0,0) \put(0,0){\epsfxsize =250pt \epsffile{dibujos/fige07.eps}} \end{picture}\\ Figure 14.7 \end{figure}

If, rather than use both the constant order and the number sequence in labeling cards, we restrict ourselves to the constant order, we will easily be able to construct any card flexagon of order $N$ from a set of cards numbered from 1 to $N$. This will include flexagons with all possible combinations of all kinds of cycles. Which of the possible permutations of the $N$ cards are we able to use? Or can we shuffle the cards, and have any random constant order make a flexagon? How many possible flexagons of order $N$ can we make? These questions lead us on, away from the card flexagons and the problems of class, and open up a whole new field of inquiry.

What requirements must we satisfy to build a flexagon? This is the question that lies at the bottom of all the problems now before us.

next up previous contents
Next: Requirements Up: Playing Cards Previous: C. Pat Structure System.   Contents
Pedro 2001-08-22