 |
 |
The basic objects are labelled de Bruijn diagrams, whose properties have been studied both numerically and symbolically. One of the first things to be done with the evolution-labelled diagram is to decompose its matrix into a sum of matrices according to the evolution. The uniform multiplicity principle will require each one of these fragments to be stochastic, as well as requiring minimum variance for the second moment matrix.
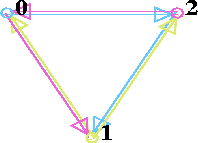
Consultation of the evolution table shown in Figure 4 reveals three de Bruijn fragments,
![\begin{displaymath}\begin{array}{rclrclrcl}
A & = &
\left[\begin{array}{ccc} 1...
... 1 & 0\\ 0 & 0 & 0\\ 1 & 0 & 1 \end{array}\right],
\end{array} \end{displaymath}](img98.gif) |
(32) |
| D | = | ![$\displaystyle \left[\begin{array}{ccc} 1 & 1 & 1\\ 1 & 1 & 1\\ 1 & 1 & 1 \end{array}\right],$](img99.gif) |
(33) |
The table of powers for the individual fragments is
![\begin{displaymath}\begin{array}{c\vert ccc}
{\rm power} & A & B & C \\ \hline ...
... 0 & 0\\ 0 & 0 & 0\\ 1 & 1 & 1 \end{array}\right] \end{array}, \end{displaymath}](img100.gif)
The fact that all these matrices are idempotent, a very suggestive relation which was noticed empirically while working out some particular examples. Inasmuch as it is not a common property of matrices, but central to understanding reversibility, it is worth exploring its origins in the algebraic properties of the connection matrices.
We can begin with one immediate consequence of the uniform multiplicity theorem by introducing the vector ![]() defined by
defined by
| = | ![$\displaystyle \left[ \begin{array}{c} 1 \\ 1 \\ \cdots \\ 1 \end{array} \right]$](img103.gif) |
(34) |
| = | (35) |
| = | (36) |
| = | (37) | ||
| = | (38) | ||
| = | (39) |
Common sense argues that the eigenvalues should not be greated than 1, just because small matrices cannot have large eigenvalues. If a connection matrix had an eigenvalue greater than 1 in absolute value, its powers would have ever greater eigenvalues, and eventually require at least one large Gerschgorin disk to hold them. That would imply some large matrix elements to provide an ample radius, which would go against the fact that the connection matrix only has positive (or zero) integer elements, and their sum is fixed, whatever the power, at the uniform value of the multiplicity.
There is then a question of possible eigenvalues in the range between zero and one, for which the answer seems to be that between these limits it would be hard to maintain the constancy of the ancestor count with all the different combinations of powers and eigenvalues that would be found. Beginning with Sylvester's formula in its full generality,
| f(M) | = | 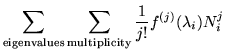 |
(40) |
| = | 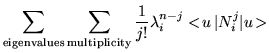 |
(41) |
By varying the power n, we have a series of equations which relate the inner product of a constant vector whose components are the matrix elements of the idempotents and nilpotents, and a series of vectors whose components depend on varying powers of a fixed set of eigenvectors. Up to a certain point such a relationship may be feasible, but if it continues there must be restrictions which need to be examined. Of course, if different powers of the ![]() 's were equal, only possible for
's were equal, only possible for
![]() or
or
![]() ,
there would be no problem, and all such matrices would be idempotent or nilpotent.
,
there would be no problem, and all such matrices would be idempotent or nilpotent.