 |
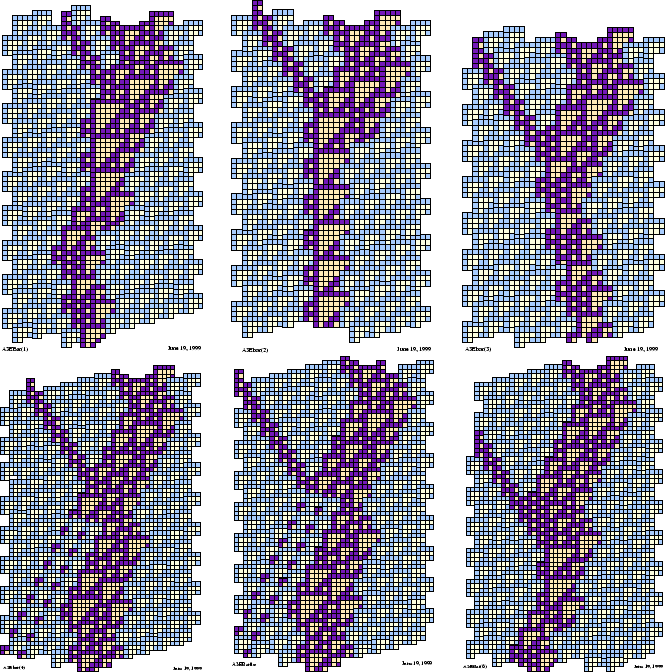
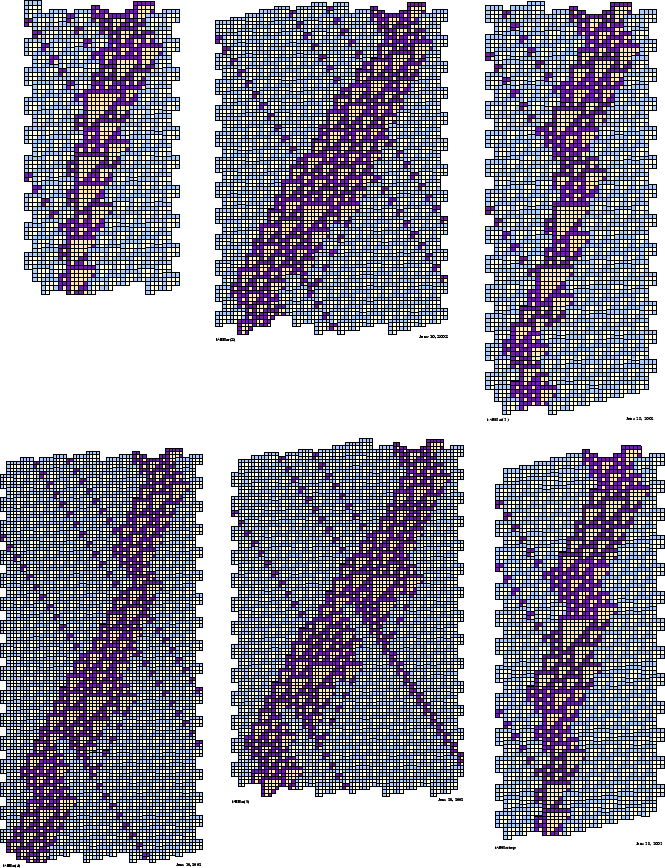
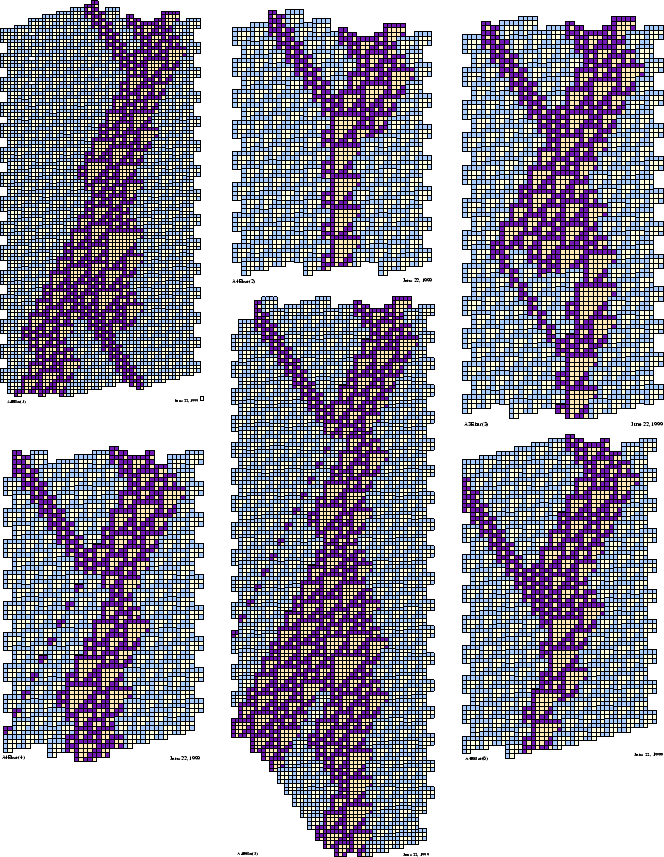
Although collisions with A gliders are not central to the demonstrtation that Rule 110 is universal, their presence is felt in certain critical places,
The most important is the flow of A tetramers coming in from the West, whose purpose is to stop the solitonic EBar gliders which have traversed all the C2 groupings in the static part of the Cyclic Tag System. But the tetramers do allow some spurious EBars to ride off into the sunset as a sort of counterflow to the tetramers. That the two possibilities exist and that their operations are so nicely synchronized is both a marvel and a source of instability.
|
Table 1 summarizes some of the An-EBar collisions. Of course, collisions with A gliders can become quite complicated, given the variability of spacing between them. If they are well enough separated, the groups collide individually and the result is cummulative. Otherwise different combinations are likely to give a variety of results.
In the simplest combination, several T1's stick together; these are called polymers, with prefixes such as monomer, dimer, trimer, ..., designating the number of T1's.
Nearly as simple are arrangements which separates monomers by single ether tiles; they are called polyads, following the sequence monad, dyad, ... .
Table 1 contains an additional column labelled ``triplet,'' which is not a generic term but refers instead to one specific combination which forms part of the Cyclic Tag System. For this glider, the spacing between monomers is 2, 5.
On the next three pages, all the six possible collisions at different aspects are shown for the three most important classes of A-EBar collisions participating in the Cyclic Tag System. Of these, not all are realized, but it is useful to know what fraction of collisions was useful, and their exact configuration.
 |
 |
 |