Siguiente: Clases de problemas Un nivel arriba: Ordenes de funciones Anterior: Funciones polilogarítmicas

Límites inferiores

Recordemos aquí la definición de límites inferiores. Sea $f:R \rightarrow R$ una función real. Entonces

$$\liminf_{n\rightarrow +\infty}f(x)=y_0\;\Leftrightarrow\; \lim_{n\rightarrow +\infty} \mathop{\rm Inf}_{x\geq n} f(x)=y_0,$$

es decir, si para cualquier 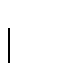$\epsilon>0$ existe 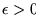$N_{\epsilon}$ tal que

$$n>N_{\epsilon}\ \Rightarrow\ \left\vert y_0-\mathop{\rm Inf}_{x\geq n} f(x)\right\vert<\epsilon.$$