Relativistic Systems

A final class of problems is composed of those involving relativistic motion, of which the most important member is Dirac's equation for an electron moving in a Coulomb potential. The most accurate analyses of the hydrogenic spectra show a fine structure, which is generally recognized as being relativistic in origin, which may be ascribed to the relativistic mass change of the electron as it moves from aphelion to perihelion with increasing velocity when one thinks in terms of the classical picture. In fact, Sommerfeld (111) had already in 1916 applied the old quantum mechanics according to the Bohr quantization rules to obtain a fine-structure formula which coincided not only with the experimental observations, but also with the corresponding formula obtained over a decade later from Dirac's relativistic wave mechanical theory. The coincidence has always been considered rather remarkable, because the old quantum mechanics begins to break down rather badly when it has to treat many-body and relativistic problems. The confusion is no doubt due to the fact that Dirac's equation, as well as its rival, the Klein-Gordon equation, involves several rather distinct phenomena in a way which makes the mixture rather obscure. On the one hand, relativistic effects occur, but it would seem that these enter predominantly through the dependence of mass on velocity, although one should not slight the powerful influence of the requirements of Lorentz invariance and covariance. Rather independently of this, it seems that one cannot escape the necessity of using a multiple-component wave equation, or, in other words, a spinor field, to describe particles such as the electron. Relativistic considerations enter to determine the number of spinor components, and their transformation properties, but do not of themselves require the occurrence of a multicomponent wave function.

To see how relativistic effects enter in at the classical level, it is instructive to review Sommerfeld's derivation of the fine structure formula, phrased in Hamiltonian terms as it is done in Born's The Mechanics of the Atom (112). The relativistic Hamiltonian for the Coulomb problem is

$$\begin{eqnarray*} {\cal H} & = & m \left(\left[ 1 + \frac{1}{2m}(p_x^2+p_y^2+p_z^2)\right]^{1/2} - 1\right) - \frac{Ze^2}{r}, \end{eqnarray*}$$

which is rather an awkward expression to use because of the occurrence of the square root of the momentum. For example, in the Hamilton-Jacobi equation, the derivatives of the principal function would be entangled in a manner which would make solution of the equation difficult. For this reason it is convenient to perform the algebraic manipulations necessary to remove the radicals, at the price of obtaining an implicit dependence of the resulting expression on the energy, $W$. We might call the resulting expressions the ``squared'' Hamiltonian:

$$\begin{eqnarray*} \frac{1}{2m}(p_x^2+p_y^2+p_x^2) & = & W + \frac{Ze^2}{r} + \frac{1}{2m}\left(W+\frac{Ze^2}{r}\right)^2, \end{eqnarray*}$$

Nevertheless, when the time-dependence has been removed from the Hamilton-Jacobi equation through the introduction of the energy constant W, the resulting expression bears many resemblances to the nonrelativistic form of the hydrogenic Hamiltonian. Apart from a renormalization of the energy and of the electric charge arising from the implicit dependence on the energy constant which Sommerfeld noted as the relativistic modification of circular orbits, the principal new feature is the occurrence of a centrifugal potential $Z^2e^4/2mr^2$, which is responsible for the orbital precession.

If one's only interest is in studying hydrogen atoms, one is likely to have to accept the ruination of the accidental degeneracy of the hydrogen atom thereby occasioned, since the Runge vector can no longer be a constant of the motion. However, if one's interest is in studying accidentally degenerate systems, irrespective of their physical reality, it is clear that the degeneracy may be restored by canceling the centrifugal potential. In fact this is the way in which we proceeded earlier in producing a degenerate version of the magnetic monopole problem.

Here, however, one's freedom is more circumscribed, because the original Hamiltonian ought to be adjusted, and not the equivalent ``squared'' Hamiltonian. Sadly, the addition of a canceling centrifugal potential in the original Hamiltonian will produce inverse third and fourth powers of the radius as well as the inverse-square potential destined to cancel the term of relativistic origin. An attempt to cancel these new terms in their turn will lead to an infinite series, from which it does not appear possible to exclude an energy dependence. Alternatively we may have recourse to a vector potential to effect the desired adjustment. This potential must have a length equal to the centrifugal term, and be orthogonal to the momentum if no residual velocity-dependent terms are to appear; such a choice is $A = Ze\hat\ell/r$, where $\hat \ell$ is a unit vector in the direction of the angular momentum. The resulting Hamiltonian,

$$\begin{eqnarray*} {\cal H} & = & m \left( \left[ 1 + \frac{1}{2m} \left({\bf p}... ...t{\ell}}{r} \right)^2 \right]^{1/2} - 1 \right) -\frac{Ze^2}{r}, \end{eqnarray*}$$

is thus one whose relativistic orbits, including a precession due to the relativistic mass change, are just the Keplerian ellipses, although there remains a renormalization of energy and nuclear charge. It is therefore a relativistic system with the $O(4)$ symmetry associated with the nonreiativistic hydrogen atom.

As for the hydrogen atom itself, a proper understanding had to await Dirac's equation, whose symmetry properties unforunately seem to have remained obscure long after its other characteristics had become established. This has been due to the fact that Dirac's equation is in reality a very complex structure, whose properties have only gradually unfolded, even with a lapse of 40 years. When it was first enunciated, it showed so many unusual and paradoxical aspects, that it was hardly possible to know where to begin with its interpretation. Its acceptance, aside from the aesthetic aspect of its being a relativistically covariant equation, rested on its success in predicting the electron spin, and its yielding the experimentally acceptable value for the fine structure of the hydrogen spectrum. Its explanation of the electron spin was an outstanding accomplishment, for this quantity bad seemingly come from nowhere, yet demanded to be taken into account in the interpretation of atomic spectra. Its ability to predict the fine structure correctly was no less impressive, for the failure in precisely this aspect had discredited Schrödinger's original relativistic wave equation, which has come to be called the Klein-Gordon equation, and had inspired Dirac's search for a more adequate relativistic wave equation in the first place, as well as having impeded Schrödinger's original formulation of wave mechanics.

In spite of its impressive and unfailing success in predicting the energy levels and probability distributions for numerous quantum mechanical systems, one began to encounter philosophical and practical difficulties with the Dirac equation almost at once. Part of the complexity of the Dirac equation is due to the fact that it is a first-order and not a second-order differential equation. Group invariants as applied to quantum mechanical problems are generally quadratic in the coordinates and momenta; we think of such things as the square of the radius, or the kinetic energy operator which is the square of the momentum vector, in the case of the rotation group, or the form $(r^2-t^2)$ preserved by the Lorentz group. Such expressions are readily converted into differential operators, whereas such functions of them as their square root are not. Moreover, the Dirac Hamiltonian derives its fame precisely from not being the square root of an invariant operator expression, but rather from being a hypercomplex operator, free of radicals, whose square results in such an invariant expression. Herein lies the hidden assumption of a multicomponent wave function, and the eventual complication that along with the infinitesimal transformations of space-time operators there must be incorporated operators mixing the components, if one hopes to produce invariant expressions. Such behavior is characteristic of any field theory, and we should recollect that even the Maxwell equations do notlead to spherical symmetry and conservation of angular momentum until account is taken of the angular momentum which may reside in the field itself.

Dirac therefore started from the relativistic energy of a free particle

$${\bf E}^2 = {\bf p}^2 + m^2$$

and postulated that there existed a hypercomplex operator of the form

$${\cal H} = {\bf\alpha} \cdot {\bf p} + \beta m ,$$

the square of which would result in the ordinary expression for the energy. To obtain such a result one must require that the operators $\alpha_i$, satisfy the relationships

$$\begin{eqnarray*} \alpha_i \alpha_k + \alpha_k \alpha_i & = & 2 \delta_{ik} , \\ \alpha_i \beta + \beta \alpha_i & = & 0 , \\ \beta^2 & = & 1. \end{eqnarray*}$$

In other words, it is necessary to have a set of four anticommuting operators, whose squares are unity. The representation theory of hypercomplex numbers shows that there is only one faithful irreducible matrix representation of such a set of operators, and that this representation requires the use of 4 x 4 matrices - the so-called Dirac matrices. As a result the number of components of an irreducible wave equation is likewise four, a result depending on the requirements for a set of four mutually anticommuting matrices and thus only very indirectly related to the four dimensionality of Minkcwskian space-time.

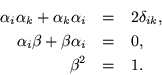
One ought to take note of several features of the assumptions involved in the formulation of the Dirac equation. The decision has been made to work with a multicomponent wave equation, and moreover that it is to assume a Hamiltonian form through the relativistic formula for the energy of a free particle. However, the operator to be used as a Hamiltonian is one whose square yields the relativistic energy, squared, in order that it itself may depend linearly on the momentum. This necessitates the intrusion of anticommuting operators; but there is an explicit assumption that the space-time operators such as the position and momentum, and the hypercomplex anticommuting operators, are cleanly separated, as it were. In other words, we are assuming that the use of a multicomponent wave function, with the space-time operators, and especially the momentum operators, acting on the various components of the wave function individually is an adequate way to avoid the square-root operators which would occur in a more straightforward transcription of the relativistic Hamiltonian into operator form. That such an arrangement actually bears fruit must be regarded as really rather remarkable, but at the same time one must clearly understand that there are two effects involved. One is that the equation is a proper relativistic equation, and so must be expected to take relativistic effects into account as an automatic consequence of its formulation. However, the other, equally important hypothesis is that of a multicomponent wave function.

Whatever the philosophical or metaphysical origin of the Dirac equation, once its usage is agreed upon, it is nothing but a set of first-order linear differential equations, to which the ordinary mathematical reasoning concerning symmetry and invariance must apply, just as any other properties of the solutions might be discussed. In this regard, the first observation which may be made is that this set of equations is not even rotationally or reflectionally invariant. From the original Hamiltonian ${\cal H} = [\alpha \cdot {\bf p} + \beta m ]$ inversion in the origin produces ${\cal H} = [-\alpha \cdot {\bf p} + \beta m ]$ while a rotation produces the Hamiltonian ${\cal H} = [\alpha \cdot {\bf p}' + \beta m ]$, where ${\bf p}' = R({\bf p})$ is the rotated momentum and therefore most certainly a different vector from the original.

Since one has the intention of using Hamiltonian mechanics in its accepted form, albeit in a formal way, and even though this Hamiltonian is now thought of as a hypercomplex number, it is convenient to define the orbital angular momentum in terms of the canonical momentum in the accustomed way,

$${\bf L} = {\bf r} \times {\bf p},$$

and to calculate its commutator with respect to the Dirac Hamiltonian. As is to be expected from the lack of manifest spherical symmetry of the Dirac Hamiltonian, one finds at once that the orbital angular momentum is not a constant of the motion; in fact its derivative is $-i\alpha \times {\bf p}$. After a certain amount of trial and error it is readily enough found that there is another ``vectorial'' quantity

$${\bf\sigma} = (\sigma_x, \sigma_y, \sigma_z)$$

$({\bf\alpha} = \rho_1 {\bf\sigma}$, in Dirac's notation) whose time derivative is the negative of that of the orbital angular momentum, justifying the introduction of the ``total'' angular momentum

$${\bf J} = {\bf L} + \mbox{$\frac{1}{2}$} {\bf\sigma}$$

as a constant of the motion, and referring to this other quantity as the ``spin'' angular momentum. Historically, its discovery was no great accident, for the electron spin was well known, and one of the major successes of the Dirac equation was just the fact that its inclusion in the conserved total angular momentum was imperative. Mathematically speaking, it is seen that the spin is a direct consequence of the multicomponent wave function, for the simple reason that a rotation of a wave not only alters the way in which the wave components depend on the coordinates of the space, but it also reorganizes the way in which they are recognized as components belonging to the various coordinates. The rotation of coordinates is done by the orbital angular momentum, while the reorganization of the components is done by the spin angular momentum. In this way a Dirac particle acquires an ``intrinsic'' angular momentum, but it must be understood that the spin angular momentum exists exclusively to relabel the four components of the multicomponent Dirac wave function. Since the Dirac Hamiltonian has the formal structure of an inner product, one can also understand the symmetry in terms of a simultaneous rotation in the two spaces joined by the inner product.

Thus the spin of the Dirac electron, while indirectly of relativistic origin, is primarily a consequence of the use of a multicomponent wave function. Galindo and Sánchez del Río (113) have emphasized this point by showing that a multicomponent Galilean invariant theory can also be constructed, in which a spin nevertheless makes its appearance. Lévy-Leblond (114) has examined such a theory exhaustively. The secondary effects of relativity, which have their Galilean counterparts, and which in fact do influence the spin, have to do with the number of components required for the spin functions, which depends on the dimension of Minkowski space. In turn, this determines the precise numerical value of the spin, as well as the transformation properties of the spinors with respect to rotations, or even more general Lorentz transformations.