B. The Sign Sequence Sums

Now that ``class'' has dissolved out from under us, the sign sequence can no longer be a simple string of signed numbers; we must now indicate the central angle between the hinges of each leaf. How this is done is irrelevant; the point is that this is the new meaning we must assign each of the terms in the sequence. The real problem, however, arises when we try to use the sign sequence.

Until now, we have adhered to a basic rule of flexagon building which states that the sum, $D$, of the terms in the sign sequence must be evenly divisible by the class; or if we choose to speak in terms of symbols, that $D\equiv 0 \;( \mbox{mod } K)\equiv 0^{\mbox{o}} \; (\mbox{mod } 360^{\mbox{o}} )$. The physical interpretation of this rule is that, when a unit is folded up with one leaf upon the other, the hinges entering and leaving this unit pat will coincide.

It is not difficult to see why this rule has always held, heretofore. All previous flexagons were produced by the slitting process, or, in the sign sequence, by replacing a given sign with a group of $K-1$ opposite signs (for $K>3$, incomplete cycles may be viewed in this case as deletions of complete cycles. Since deletion clearly does not change the sign sequence sum, incomplete cycles pose no difficulty.) Hence $D$ remained constant for a given $G=K$. Considering only the one-cycle flexagon of cycle $G$, therefore, we see that its sign sequence is composed of $G=K$ like signs, so that

$$\begin{eqnarray*} D & = & K x \mbox{ (unit sign) } = K x \frac {360^{\mbox{o}} ... ...d } K) \; \equiv \;0^{\mbox{o}} ( \mbox{ mod } 360^{\mbox{o}} ). \end{eqnarray*}$$

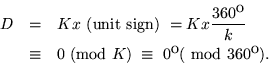
However, in the heterocyclic flexagons made from regular polygonal leaves, slitting did not replace a sign by $K-1$ opposite signs; slitting replaced one sign in the old cycle, where $G=m$, say, by $n-1$ opposite signs, where $n=G_{\mbox{new cycle}}\neq m$. Hence, the sign sequence sums for this slitting are unequal:

$$\begin{array}{rlrl} D_{m \mbox{ cycle}}\; = & 1 \;x \;\mbox{... ...pi) & \equiv &\frac {2 \, \pi}n(\mbox{mod } 2\,\pi) \end{array}$$

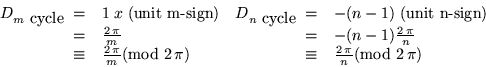
At this point we will either force the difference $\frac 1 m - \frac 1 n = D\; (\mbox{mod } 360^{\mbox{o}} )$ to become congruent to zero, by adding in other cycles or by using one of the techniques for destroying class distinctions given previously, or we will leave $D\neq 0 \;\mbox{mod } 360^{\mbox{o}}$.

In the latter case, when the flexagon unit is folded together into a unit pat, as it is, for example, in flexing, the incoming and outgoing hinges will not coincide, and the ability to flex will depend only on the magnitude of $D$ and the elasticity of the materials. Of course, if we wish to abandon class altogether, the sign sequence may be juggled around at will, and we need only make the angles have any values that will satisfy the rule. If we wish to use regular polygons as leaves, $D$, the sum of all discrepancies such as $(\frac 1 m - \frac 1 n )$, must be computed. In doing this, the terms representing a given cycle must always be given the same sign in the difference (whenever $\frac 1 n$ occurs it will always be preceded by a minus sign; $\frac 1 m$, never). When $D\equiv 0^{\mbox{o}} \;(\mbox{mod } 2\pi)$, the flexagon will operate without difficulty (except, of course, the difficulty encountered in overlapping assorted polygons). 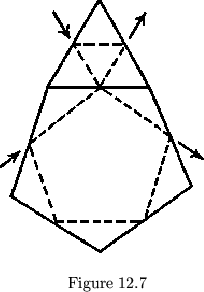It is interesting to note that, as predicted, $D$ for the flexagon shown in fig. 12.7 is uninfluenced by the presence of the triangular cycle, but is also uninfluenced by the pentagonal cycles. The computation for this flexagon goes:

$$\frac D {2\, \pi} \equiv \sum (\mbox{differences}) \;(\mbox{mod } 1)$$

$$\begin{eqnarray*} \sum (\mbox{differences}) & = & (\frac 1 3 - \frac 1 4)+(\frac... ...mbox{mod } 1)\\ D & \equiv & \frac \pi 2 ( \mbox{mod } 2\,\pi) \end{eqnarray*}$$

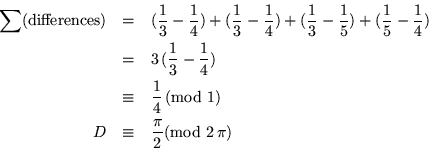
Using this method, the feasibility of a given flexagon may be guessed almost at once. Thus $D$, for the flexagon shown in fig. 12.8, is clearly quite near to zero, since the difference between one-fourth, and one-fifth is almost the same as that between one-third and one-fourth. Or, actually doing the computation, $\sum \mbox{(differences)} = (\frac 1 5 - \frac 1 4) + (\frac 1 3 - \frac 1 4) = \frac 1 {30}$, and $D=12^{\mbox{o}} (\mbox{mod }360^{\mbox{o}} )$, which is close enough to allow flexing with reasonably elastic materials.

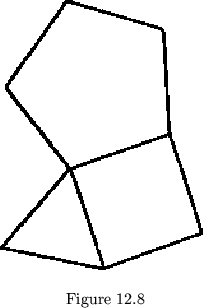
A graphic demonstration of these angle differences is given when the hinge network is constructed. Where two polygons of different types meet, the network lines must be bent (See fig. 12.7).