


Next: boundary conditions and the
Up: Uniform strings
Previous: eigenvalues and eigenvectors
Contents
This expression simplifies drastically when 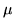 is written as an exponential,
is written as an exponential,
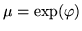 , and it is supposed that
, and it is supposed that 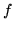 is an n
is an n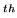 power:
power:
Still further simplification awaits: First take exponential factors out of the denominators
and then recognize that the 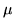 's are reciprocals, so their logarithms are negatives. The matrix
's are reciprocals, so their logarithms are negatives. The matrix 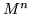 is accordingly a function of a single angle and with common denominators the sum becomes a difference:
is accordingly a function of a single angle and with common denominators the sum becomes a difference:
Moreover, the matrix elements, with the participation of the obstreperous denominators, are nothing other than Tchebycheff polynomialsTchebycheff polynomials of the second kind (with imaginary argument),
the denominator 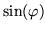 generally serving to make derivatives turn out right.
generally serving to make derivatives turn out right.
Some patience with trigonometric identities will confirm
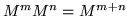 , which must be so because of the context in which it occurs.
, which must be so because of the context in which it occurs.
Slight additional trigonometrical transformation (writing s and c for sinh and cosh to fit the formula onto the page) produces
The algebra of the wave matrices having been attended to, the determination of the eigenvalues of the dynamical matrix depends on choosing appropriate boundary conditions for a relationship such as

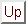


Next: boundary conditions and the
Up: Uniform strings
Previous: eigenvalues and eigenvectors
Contents
Pedro Hernandez
2004-02-28
![]() is written as an exponential,
is written as an exponential,
![]() , and it is supposed that
, and it is supposed that ![]() is an n
is an n![]() power:
power:
![\begin{eqnarray*}
M^n & = &
\frac{\exp(n \varphi_+)}{\exp(2\varphi_+) - 1}
...
...
\exp((n+1)\varphi_-) & \exp(n\varphi_-)
\end{array} \right]
\end{eqnarray*}](img448.gif)
![\begin{eqnarray*}
M^n & = &
\frac{1}{\exp( \varphi_+) - \exp(- \varphi_+)}
...
...n \varphi_-) & - \exp((n-1) \varphi_-)
\end{array} \right] \\
\end{eqnarray*}](img449.gif)
![]() , which must be so because of the context in which it occurs.
, which must be so because of the context in which it occurs.
![\begin{eqnarray*}
M^n & = & \frac{1}{\sinh(\varphi)} \left[ \begin{array}{cc}
...
...) & -1 \\
1 & - \cosh(\varphi)
\end{array} \right] \right).
\end{eqnarray*}](img456.gif)