Any tubulating flexagon (that is, one with exposed tubulations, also
called an incomplete flexagon) may be made by deleting a given number of
sides from a certain complete flexagon of order ![]() where
where ![]() is the
order of the tubulating flexagon and
is the
order of the tubulating flexagon and ![]() is the order of the flex involved
in a tubulation. What we want to know now is what effect on the plan
this tubulation has. Let us take for an example a complete proper
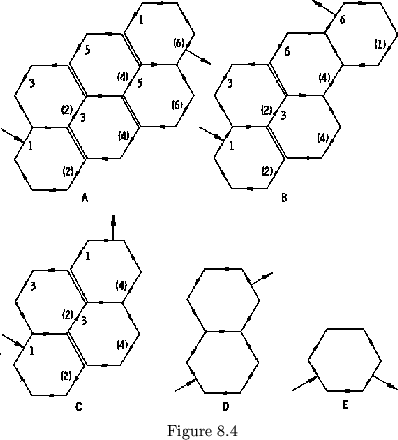
hexaflexagon of order 6 (see figure 8.4a). If we wish to delete a side,
say side 5, we fold the two 5's in the plan together. When we do this,
we find that the hinge difference across the double leaf is +2 (or
is the order of the flex involved
in a tubulation. What we want to know now is what effect on the plan
this tubulation has. Let us take for an example a complete proper
hexaflexagon of order 6 (see figure 8.4a). If we wish to delete a side,
say side 5, we fold the two 5's in the plan together. When we do this,
we find that the hinge difference across the double leaf is +2 (or ![]() )
(See figure 8.4b). Now if we want to delete side 6, fold the 6's together,
and the total hinge difference across the triple leaf is
)
(See figure 8.4b). Now if we want to delete side 6, fold the 6's together,
and the total hinge difference across the triple leaf is ![]() (see figure
8.4c). If we were to take two hexagonal leaves, each with a hinge
difference of +2, (see figure 8.4 d,e), the total hinge difference when
the leaves are folded together would be +4 or
(see figure
8.4c). If we were to take two hexagonal leaves, each with a hinge
difference of +2, (see figure 8.4 d,e), the total hinge difference when
the leaves are folded together would be +4 or ![]() . In general, whenever we
fold together two leaves with hinge differences respectively of
. In general, whenever we
fold together two leaves with hinge differences respectively of ![]() and
and
![]() , the total hinge difference will be
, the total hinge difference will be ![]() or
or ![]() . To produce an
exposed
. To produce an
exposed ![]() flex, we must delete
flex, we must delete
![]() leaves from a complete proper pat, but since each of these
leaves has a hinge difference of 1, the total hinge difference across the
leaf resulting from the deletions must be
leaves from a complete proper pat, but since each of these
leaves has a hinge difference of 1, the total hinge difference across the
leaf resulting from the deletions must be ![]() . Therefore, in the
plan, an
. Therefore, in the
plan, an ![]() flex requires a leaf with a hinge difference of
flex requires a leaf with a hinge difference of ![]() .
.
Example:
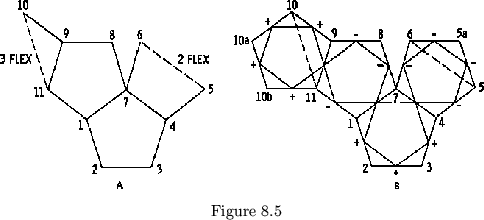
Find the plan for the flexagon with the map as shown in figure 8.5a. The method of attack for this problem is to view the tubulating flexagon as a product of the deletion of sides from the flexagon with the map as shown in figure 8.5b. The sign and number sequence for this flexagon will be:
| + | + | + | - | - | - | - | - | - | + | + | + | - | - | + |
| (1) | 3 | (3) | 7 | (5a) | 5a | (4) | 1 | (9) | 10a | (l0a) | 11 | (8) | 8 | (1) |
| 2 | (2) | 4 | (6) | 6 | (5) | 5 | (11) | 10 | (10) | 10b | (10b) | 9 | (7) | 2 |
Now if we delete side ![]() , the hinge difference across the new leaf will
be
, the hinge difference across the new leaf will
be ![]() or
or ![]() . The process of deleting this side will turn upside down
that part of the plan from 5 on:
. The process of deleting this side will turn upside down
that part of the plan from 5 on:
| + | + | + | - | - | - | + | + | + | + | - | - | + | |
| (1) | 3 | (3) | 7 | (5) | 5 | (11) | 10 | (10) | 10b | (l0b) | 9 | (7) | (2) |
| 2 | (2) | 4 | (6) | 6 | (4) | 1 | (9) | 10a | (10a) | 11 | (8) | 8 | (1) |
The sides ![]() and
and ![]() will be deleted next. The hinge difference across
this new leaf will be
will be deleted next. The hinge difference across
this new leaf will be
![]()
![]() or
or
![]() ,
and since there are two deletions
in this operation, the part of the plan beyond
,
and since there are two deletions
in this operation, the part of the plan beyond ![]() will be flipped over and then flipped back:
will be flipped over and then flipped back:
| + | + | + | - | - | - | + | - | - | + | ||
| (1) | 3 | (3) | 7 | (5) | 5 | (11) | 10 | (10) | 9 | (7) | (2) |
| 2 | (2) | 4 | (6) | 6 | (4) | 1 | (9) | 11 | (8) | 8 | (1) |
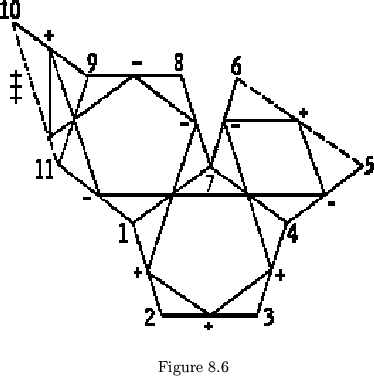
A shortcut that eliminates all of this complex figuring is to draw a polygon system including all tubulations (see figure 8.6). The points in the polygon system which touch tubulations are given values according to the order of the flex the tubulation is, and according to whether the turn is positive or negative. This method produces identical results with the previously mentioned method.