relation to complex numbers

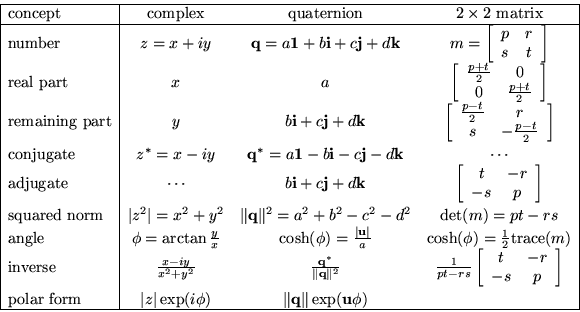
This collection of arithmetic curiosities can be summarized by referring to the original objective in introducing quaternions, to be able to rotate vectors. Rotating implies angles, and suggests polar coordinates. In the familiar environment of complex numbers, the norm is the radius and the argument is the angle measured from the real axis. Real and imaginary refer to cartesian coordinates. The following table draws analogies between complex numbers, Hamilton's quaternions, and the $2 \times 2$ matrices.

$$\begin{array}{\vert l\vert ccc\vert} \hline {\rm concept}&... ... \Vert{\bf q}\Vert\exp({\bf u}\phi) & \\ \hline \end{array}$$