 |
Having introduced the study of symmetry by using the example of a cyclic chain, which has both shift and reflection symmetry, we could finish by examining the symmetry group, its irreducible representations, and their effect on the dynamical matrix. If the particles were located at the vertices of a regular polygon, the symmetry group could be interpreted as the set of rotations and reflections of that geometric figure.
Symmetry of an n-gon has two generators, a rotation ![]() for which
for which ![]() ,
and a reflection
,
and a reflection ![]() for which
for which ![]() , for which
, for which ![]() . Groups are often defined by listing a set of generators and a set of relations, three in this case. The full group table is supposed to be deduced from this information, but that is not always possible, for reasons which lead deeply into the theory of recursive functions. Fortunately the table for the symmetry of polygons is easily calculated, resulting there are only
. Groups are often defined by listing a set of generators and a set of relations, three in this case. The full group table is supposed to be deduced from this information, but that is not always possible, for reasons which lead deeply into the theory of recursive functions. Fortunately the table for the symmetry of polygons is easily calculated, resulting there are only ![]() symmetries, of the form
symmetries, of the form
![]() with the rule of multiplication
with the rule of multiplication
If ![]() has divisors, both cyclic and dihedral groups contain subgroups whose orders are the divisors, generated by the powers of
has divisors, both cyclic and dihedral groups contain subgroups whose orders are the divisors, generated by the powers of ![]() complementary to the divisor. Thus both
complementary to the divisor. Thus both ![]() generated by
generated by ![]() and
and ![]() , and
, and ![]() , generated by
, generated by ![]() and
and ![]() are subgroups of
are subgroups of ![]() .
.
In addition, any of the products ![]() generate subgroups of order
generate subgroups of order ![]() , since
, since
![]() . The generators of these subgroups are reflections, and come in two forms. If
. The generators of these subgroups are reflections, and come in two forms. If ![]() is even, reflections in lines passing through opposite vertices are not the same as reflections in perpendicular bisectors of opposite edges. In fact, the vertex splitting reflections constitute a class, the edge-splitting reflections another.
is even, reflections in lines passing through opposite vertices are not the same as reflections in perpendicular bisectors of opposite edges. In fact, the vertex splitting reflections constitute a class, the edge-splitting reflections another.
On the other hand, when ![]() is odd, reflections bisect one vertex angle and one opposite edge each, so there is no distinction and all the reflections make up one single class.
is odd, reflections bisect one vertex angle and one opposite edge each, so there is no distinction and all the reflections make up one single class.
The subgroups mentioned are all there are. Each defines a family of cosets, but only the cyclic subgroups are normal.
In enumerating the classes, we have just seen that the reflections form either one single class or two distinct classes, according to the parity of the n-gon. Since all the rotations commute, the size of one of their classes depends on the reflections, which map rotations into their inverses. Therefore, again according to parity, there are ![]() or
or ![]() classes of rotations. When
classes of rotations. When ![]() is even,
is even,
![]() , so it and
, so it and ![]() each sit in a single class, for a total of 2. The other
each sit in a single class, for a total of 2. The other ![]() rotations pair up in
rotations pair up in ![]() classes, for the total stated. When
classes, for the total stated. When ![]() is odd, only the identity is self-conjugate, so the prospective number of classes is reduced by 1.
is odd, only the identity is self-conjugate, so the prospective number of classes is reduced by 1.
If the n-gon is a plane figure, we already have a matrix representation with
![]() matrices:
matrices:
![\begin{eqnarray*}
D(S) & = & \left[ \begin{array}{cc} \cos \theta & - \sin \the...
...\left[ \begin{array}{cc}
0 & 1 \\ 1 & 0
\end{array} \right],
\end{eqnarray*}](img663.gif)
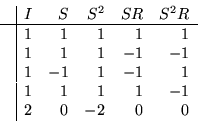
![\begin{displaymath}\begin{array}{c\vert cccccccc}
& I & S & S^2 & S^3 & R & SR...
...in{array}{cc} 1 & 0 \\ 0 & -1 \end{array} \right]
\end{array} \end{displaymath}](img664.gif)