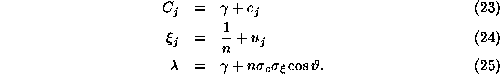The statistical theorem

Supposing that the required means and variances have been calculated, we could write

 

From our point of view, the interesting result is that

 

and that deviations of from this value are expressible in terms of the variances, both of the column sums and the eigenvector components.

The factor n in Eq. 25 may be disconcertingly large unless it is compensated by considerable uniformity in the column sums and/or in the components of the eigenvector. When either, and especially both, are small, the eigenvalues are estimated by the element sum of the matrix. Since similar estimates apply to all eigenvalues (with suitable modifications for complex eigenvectors whose components do not sum to zero), it can be seen that the orientation of the eigenvector relative to the vector of column sums distinguishes between the different eigenvalues.

---

Harold V. McIntosh
E-mail:mcintosh@servidor.unam.mx