The topological matrix of the vector subset diagram manifests some interesting structural details. It is not quite a tensor product, but the nonzero submatrices fall exactly on the locations of the nonzero elements of the scalar matrix. The submatrices are of two types, corresponding to the decomposition of the symbolic de Bruijn matrix, being derived from them through multiplication on the right by a projection onto the subset defining the row. In the matrix shown, the projector for the first row is the identity matrix, for the second it preserves components D and F, while the third goes with A and C.
Moreover a supermatrix whose diagonal submatrices are the appropriate projectors commutes with the vector subset matrix, just as it commutes with each of the two summands resulting from the symbolic decomposition of the de Bruijn matrix. Incorporation of this projector as a factor allows writing the vector matrix as a sum of two tensor products, which in turn facilitates the evaluation of powers of the vector matrix and products of its summands.
Suppose that S is the scalar subset matrix, 
the projector, 
the vector subset matrix, and that the de Bruijn matrix
D is decomposed into the sum A+B according to a criterion such
as the value of the evolved cell. Then we would have
for example the factorization below defines 
:
0.24em
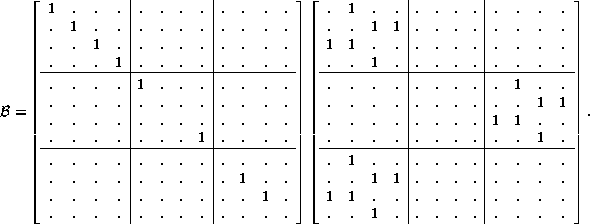
An immediate consequence of this representation is the fact that in any product of supermatrices, all the submatrices are formed from products of the two terms into which the de Bruijn matrix is decomposed, even though they may eventually have to be projected to obtain a final result. This establishes a formal relation between the methods of this section and the procedures of Erica Jen [13] or Backhouse and Carré [4].