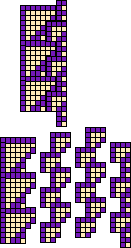
Figure 37: T6 based gliders, which actually don't move.
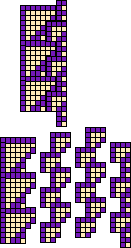
Figure 37: T6 based gliders, which actually don't move.
The C gliders are based on T6 tiles, which must be supplemented if they are to fill up the plane. Placing a T1 just under the diagonal at bottom left creates a niche for a T3 just to its right, but which is better kept separate.
Thus the first tile is the combined T1 and T6, which has a height of seven cells. The second tile has two T3's, one above and slightly to the right of the other, for a combined height of eight cells. But this pair can be stacked in a vertical column by aligning the top row of the bottom tile with the bottom row of the lower tile, once again an effective height of seven.
Another combination with a height of seven and stackable in a vertical column compatible with either of the first two tiles, is the third tile shown at the far right in Figure 37. It is built from a stack of two T1's nestled under a T3, whose staggered four-three left margin is congruent to the margin in the T3 stack.
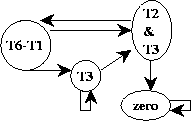
Figure 38: Sequence diagram for the tiles in C gliders.
The T6ish tiles have a left margin which is a vertical spine -- a column of
1's which extends indefinitely, which is just the left margin of s whimsical
T![]() . Meanwhile the third tile, featuring T1's, has a similar right
margin. As a result, T6's which have been connected to none or more T3's can
reconnect to another T6 to create an environment in which T6's and T3's
alternate; or they can connect to a zero half-space, from which there is no
return. In other words, a static fuse can exist.
. Meanwhile the third tile, featuring T1's, has a similar right
margin. As a result, T6's which have been connected to none or more T3's can
reconnect to another T6 to create an environment in which T6's and T3's
alternate; or they can connect to a zero half-space, from which there is no
return. In other words, a static fuse can exist.
Figure 38 summarizes the sequences which can be formed, all of period 7. The columnar form of some of the margins is to be noted; it is related to the ability to interface with a half-space of zeroes, and on a more modest scale, with any large triangle..