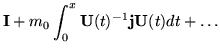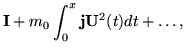


Next: Mass comparable to height
Up: Periodic Potentials in One
Previous: Dirac equation
When the mass is small or even zero, the coefficient matrix of the Dirac equation is nearly a scalar multiple of the unit antisymmetric matrix 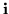 .
Accordingly solutions take the form seen in Equations (13) and (13). For the potential
.
Accordingly solutions take the form seen in Equations (13) and (13). For the potential  the results for the interval
the results for the interval 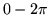 are
are
and accordingly
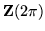 |
= |
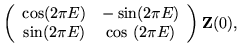 |
(18) |
half of whose trace is
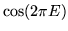 ,
independently of the strength of the potential, and even of its form so long as it is periodic. This is evident in Figure 6 and even in the contour plot of Figure 7, whose apparent structure is more likely due to the precision of the computation than to the effects of mass, height, and energy.
,
independently of the strength of the potential, and even of its form so long as it is periodic. This is evident in Figure 6 and even in the contour plot of Figure 7, whose apparent structure is more likely due to the precision of the computation than to the effects of mass, height, and energy.
Mass and energy may be separated in the Dirac equation by writing the coefficient matrix as M = A + B where
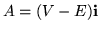 and
and
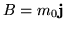 .
Factoring the solution into
.
Factoring the solution into
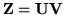 and solving
and solving
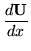 |
= |
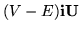 |
(19) |
to get the result already shown in Equation 18 (where the solution was called 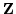 ), there remains the solution of the equation for
), there remains the solution of the equation for 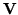 ,
,
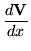 |
= |
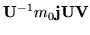 |
(20) |
When m0 is small, it is approximately solved by the so-called first Born approximation
the second form being due to the anticommutation of the quaternion matrices.
Taking into account that 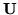 is a combination of
is a combination of 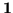 and
and 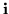 while the integral is a mixture of
while the integral is a mixture of 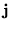 and
and 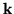 ,
changes in the dispersion relation due to a small mass will be of at least second order.
,
changes in the dispersion relation due to a small mass will be of at least second order.
Figure 6:
A perspective view of the stability function for the relativistic Mathieu potential. Mass = 0.125, nearly negligible. Strength = 1.0. The energy varies from 0.0 to 3.5, the height of the potential form 0.0 to 1.5. Negative energies give the same results as positive energies. The mass causes a slight expansion of the interval
-1.0 - 1.0 occupied by the massless half trace, something visible in the change of coloring.
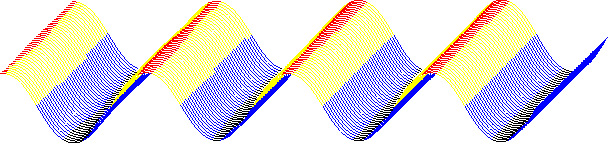 |
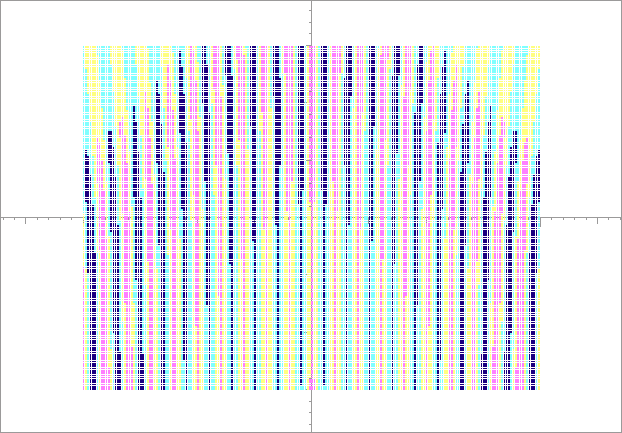
Figure 7:
Stability contours for the relativistic Mathieu potential. There is essentially no variation with respect to the height of the potential, with results very close to those for a mass of exactly zero.
 |



Next: Mass comparable to height
Up: Periodic Potentials in One
Previous: Dirac equation
Microcomputadoras
2001-01-09
![]() .
Accordingly solutions take the form seen in Equations (13) and (13). For the potential
.
Accordingly solutions take the form seen in Equations (13) and (13). For the potential ![]() the results for the interval
the results for the interval ![]() are
are
![]() and
and
![]() .
Factoring the solution into
.
Factoring the solution into
![]() and solving
and solving