Continuation in the Complex Eigenvalue Plane

The exact nature of the spectral density depends to a considerable extent on the potential employed. It would seem that some hesitation has arisen in the application of S-matrix techniques on account of a lack of familiarity with the characteristics to be expected of differing potentials. For example, square well potentials, and in general those potentials which are cut off, oscillate, or have abrupt changes, tend to have a rich assortment of complex poles near the real axis in the complex energy plane. Potentials which vary smoothly, for example those which behave as a power of the distance, may have only an essential singularity at infinity, as happens for the pure Coulomb potential. Exponentially decreasing potentials show an assortment of real poles on the second sheet, without having any strictly complex poles. It would appear that this is a phenomenon relatively familiar to acoustical or electrical engineers, for whom exponential horns have a special significance. Yet considerable consternation occurred among physicists when this potential was first encountered in S-matrix theory.

Potentials which offer definite but not insurmountable barriers to particle motion have a much better developed pole structure in the spectral density function than those which are monotonic and never rise above their asymptotic value. Potentials created by a configuration of a few fixed atomic nuclei with their Coulomb potentials do not show such behavior, but the Stark effect in atomic spectroscopy is an excellent example of a system in which, in the idealized case, the potential even drops to negative infinity at large distances. When the electric field strength is reasonably large the barrier to ionization of the system is not particularly great, giving continuum states concentrated around the perturbed energy levels, but with an appreciable level width.

Another more esoteric example is to be found in the Dirac equation, in the physically not very realistic case in which the rest mass and the binding energy of the particle are comparable. Here the Klein paradox enters into play, wherein it is found that an appreciable tunneling is possible between positive and negative energy states. The result is that increasing potentials, such as the harmonic oscillator potential, have a continuum of eigenvalues rather than the well-defined discrete spectrum which we find in the non-relativistic oscillator described by the Schrödinger equation. However, as the rest mass increases in relation to the strength of the quadratic potential well, it is found that the continuous spectrum becomes more and more concentrated, approaching more and more to a discrete spectrum in appearance.

Square well potentials, singly or in combination, afford some of the simplest examples because of the ease with which explicit solutions for either the Schrödinger equation or the Dirac equation may he formed and applied to the determination of the spectral distribution function. Moreover it is possible to arrange some very illuminating combinations such as an exponential well or a Yukawa well. A notch a finite distance from the origin was Gamow's original model for the radioactive decay of a nucleus by the emission of an alpha particle.

A clarification of the exponential well would be especially pertinent because it was the model in which the false poles of the S-matrix made their debut. With such a potential we are not too far from the result known to electrical engineers that the best impedance match between two dissimilar lines is to be had by giving the joining section the geometric mean of the two impedances which have to be matched.

One slight caution which must be observed in building up limiting approximations to potentials is that a limiting sequence of poles is likely to turn into a branch cut in the complex plane of the spectral density, giving the limiting potential ralher different properties than those of its approximations For example, cutoff potentials seem to have a different singularity structure from those which are strictly analytic Analytic continuation is a highly sensitive and unstable numerical procedure, for which relativly insignificant alterations of the continued function in one region can produce exaggerated effects in another As a result, poles near the cut line and reasonably close to the origin have a better chance of being detected, while the behavior in remoter areas is much more uncertain.

It would seem that numerical problems of this nature have held back the large-scale description of scattering processes through a Mittag-Leffler expansion of the spectral density, even when the analyticity of the m function is already conceded and the possibility of its continuation is not in doubt. Nevertheless, both the Schrödinger and the Dirac equations can be analyzed, and many general conclusions obtained, by working with carefully chosen piecewise constant potentials.

A $2\times2$ matrix notation is admirably suited for discussing one-dimensional problems. For the Schrödinger equation, we would write

$$\begin{eqnarray*}\frac{d}{dx}{\bf Z}& = & \left(\begin{array}{cc} 0 & 1 \\ V-E & 0 \end{array}\right) {\bf Z} \end{eqnarray*}$$

taking ${\bf Z}$ as a matrix containing two linearly independent solutions. By introducing the variables

$$\varphi = w(V-E)^{1/2},\ \ \sigma = (V-E)^{-1/2}$$

the solution matrix of the equation assumes the form

$$\begin{eqnarray*}{\bf Z}(w) & = & \left(\begin{array}{cc} {\rm cosh\ } \varphi ... ...\ {\rm sinh\ }\varphi & {\rm cosh\ }\varphi \end{array} \right) \end{eqnarray*}$$

In a similar manner, if we take the Dirac equation to have the form

$$\begin{eqnarray*}\frac{d}{dx}{\bf Z}& = & \left(\begin{array}{cc} 0 & m_0-(V-E) \\ m_0+(V-E) & 0 \end{array}\right) {\bf Z} \end{eqnarray*}$$

the substitutions

$$\varphi=w[m_0^2-(V-E)^2]^{1/2}, \ \ \ \sigma = \left(\frac{m_0-(V-E)}{m_0+(V-E)}\right)^{1/2}$$

lead to a solution matrix of just exactly the same form.

Multiplying together the solution matrices belonging to the constant segments is the way to resolve a piecewise constant potential, and even a good way to approximate a more arbitrary potential. Although the result can be quite explicit, increasingly cumbersome algebraic expressions arise when there are more than a very few segments. Their complexity is even more severely compounded by calculating the bracket of two solutions. Especially because the numerical behavior is easily hidden in a complicated formula, it is helpful to have some simpler estimates available.

When f represents the bounded solution, while $\psi$ and $\varphi$ are the unbounded basis solutions, an approximation may be obtained by dividing the defining equation

$$\begin{eqnarray*}f & = & \varphi + m \psi \end{eqnarray*}$$

by $\psi$. Because the left-hand side would become negligible in the limit, there remains the estimate

$$\begin{eqnarray*}m & = & -\lim_{x\rightarrow\infty}\frac{\varphi(x)}{\psi(x)}. \end{eqnarray*}$$

As no bracket is involved, it is a formula which is algebraically much simpler than the complete expression would be. However, it is only valid for limit point potentials, and not where the limit circle arises.

The asymptotic form for the solutions may already be deduced once the coefficients of the differential equation are nearing their limiting values. Regarding the coefficient matrix as constant from that point onward, the solution matrix can be factored in the form

$$\begin{eqnarray*}{\bf Z}(w) & = & \left(\begin{array}{cc} {\rm cosh\ } \varph... ...sinh\ }\varphi + D\ {\rm cosh\ } \varphi \end{array} \right) \end{eqnarray*}$$

where $\varphi$ corresponds to the width of the asymptotic segment.

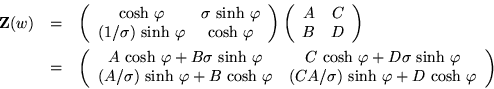
This matrix can be given a more precise appearance by defining

$$\begin{array}{ll} r = (A^2-B^2\sigma^2)^{1/2} \;, \; & \del... ...} \;, \; & \delta_2 = {\rm arctanh\ }(-D\sigma/C) \end{array}$$

and eventually,

$$\begin{eqnarray*}{\bf Z}(w) & = & \left(\begin{array}{cc} r\ {\rm cosh\ }(\va... .../\sigma)\ {\rm sinh\ }(\varphi+\delta_2) \end{array} \right). \end{eqnarray*}$$

Combining this form of the solutions with the estimate for the m function, we obtain

$$\begin{eqnarray*}m & = & -(s/r)\exp(\delta_1-\delta_1). \end{eqnarray*}$$

This is the formula which establishes the closest connection between the theory of differential equations and the S-matrix theory of quantum mechanics, by exhibiting the identity of the S-matrix and the spectral density in a way which clearly shows the assumptions involved. In one dimension, the S-matrix is a single number, whereas in general it is a matrix. However, the spectral density also generalizes to a spectral matrix when there is a system of coupled differential equations, so that the connection can be maintained quite generally.

The assumptions at stake consist in having a limit point differential equation, and having m defined for real eigenvalues Then the formula can even be used to estimate m from a purely real wave function because r and s each have simple interpretations - the asymptotic amplitudes of their wave functions Thus may be obtained the factor r_i² which is needed in the Stieltjes integral which defines the spectral distribution function.

It is interesting that m is approximated by a quotient of amplitudes rather than by the square of a single asymptotic amplitude. The implication is that the two amplitudes are reciprocals, which is the relationship by which the poles of the S-matrix on the unphysical sheet are interpreted in terms of the zeros of the complementary wave function on the physical sheet. This in turn has promoted the interpretation of real poles on the unphysical sheet as ``antibound'' states, whose ``wave functions'' grow in the worst possible way.

All these concepts need to be employed with considerable caution, because several things are being taken as equivalent which in reality only coincide under explicit but hardly all-inclusive circumstances. For example, the square of the amplitude of the finite Sturm-Liouville solutions only enters in the approximation of the spectral distribution. Additional requirements are the assumption of differentiability and a dispersion relation to arrive at the special spectral density. Finally an amplitude and the reciprocal of the complementary amplitude enter through an approximation to the m function, and define the Jost function, which is the S-matrix for one dimension.