 |
This is the flexagon. Or, rather, this is a tiny slice of the whole flexagon problem, most of which has not even been hinted at. If you enjoy the really obscure, and if you are willing to delve into some of the gory details, there are several variations on the theme ...
Flexagons need not be built of just three pairs of pats. Four pairs work just as well. With two pairs, most of the possible faces are eliminated. One pair can be flexed in theory only. Any number will do, but only three will lie flat; unless, of course, we change some angles.
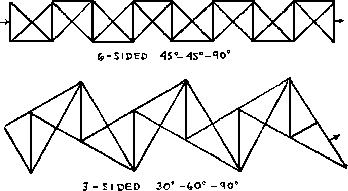
The triangles need not be equilateral, although a convenient rule is that they must overlie one another in an even stack. A pair of interesting specimens that have been built successfully are a six-sided isosceles-right- triangle flexagon of eight pats, and a three-sided 30°-60°-90° triangle flexagon of twelve pats, both of which lie flat when certain sides are exposed. These may be made from the plans shown in figure 29. The order of numbering the triangles in the plan is the same for these as for ordinary straight-plan six- and three- sided flexagons. You may figure out the details. By the way, flexagons made up from odd-shaped triangles tend to fall apart easily so that the practical limit is lowered enormously hovering just above three sides. To break another rule, flexagons need not be built of triangles. Square flexagons, called tetraflexagons, are planned and built with little more difficulty than flexagons made of triangles. Only two pairs of pats need be used. To make the simplest tetraflexagon follow the plan in figure 30. Notice the square map.
As a general example of flexagons of higher ``class'' than three; i.e., made up of polygons with more than three sides, we will build a pentaflexagon (made up of pentagons). There is a connection, which we will not bother to prove here, between the ``class'' (number of sides of the polygons in the plan) and the ``cycle'' (number of sides of the polygons in the map). They are usually the same. Thus the pentaflexagon we will build has the pentagonal map shown in figure 31. First make the Tukey triangle network by joining midpoints of sides as in figure 32. Then number the vertices as shown, and copy down the number sequence:
| 1 | 3 | 8 | 6 | 1 | ... | ||||||
| 2 | 4 | 7 | 5 | 2 | ... |
In finding the signs, there are extra directions involved, but these are merely treated in the usual way. Copying the two sequences in final form we have:
| 1 | 3 | 3 | 5 | 8 | 8 | 6 | 6 | 1 | 3 | ... |
| 2 | 2 | 4 | 4 | 1 | 7 | 7 | 5 | 2 | 2 | ... |
| R | L | R | L | L | R | L | R | R | L | ... |
To build the plan, always leave a given leaf as soon as possible on the right or left. Don't take the second side in a given direction in either case (see figure 33). The plan we make will look like figure 34, except that in the figure all the signs have been changed to show that this does not influence the final flexagon.
Two pairs of pats are needed; therefore, this sequence of pentagons will be repeated. The final flexagon, which does not lie flat, is seen in figure 35. It is not difficult to operate once you become accustomed to it, and a little experimentation with flexagons such as this produces fascinating results.