The data eventually becomes so voluminous that it seems only to be worthwhile tabulating the cycles into which the rings of length N evolve, ignoring all the transients. Below, we show one particular case, N = 16, in detail. The ring is long enough to show a variety of interesting behaviour, but yet not so long that it cannot still be readily displayed.
The notation 
describing each cycle means that the period of the
cycle is x, but that there are only y distinct phases within the
cycle. This could either mean that the original pattern has reflected
after y generations and will be completed by running through the
mirror images, or that the pattern has been translated by a certain
amount. Thus x must be a multiple of y, cycles of the form 
almost always (but not exclusively) resulting from reflection.
Sometimes an even more explicit notation, such as 16:14.7(ref) or
is required, in which the length of the ring is
shown along with an indication of the direction and distance of
displacement. Since reflectivity could be inferred if a displacement
were not shown, it is not always indicated.
First, we show the smallest numerical representatives for each of the eight distinct symmetry classes of cycles for a ring of length N=16:
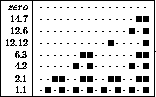
Figure: 
Rule 22 rings of length 16 have eight symmetry classes
of cycles.
Next, the eight figures which follow show a full cycle of evolution for every one of these patterns. In preparing each illustration, every line is copied twice to improve perception of the region which would otherwise be broken up by the boundary.