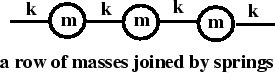Uniform strings

The tridiagonal matrix for the vibrations of a a uniform string consisting of particles of mass m connected by springs of elastic constant k, none of whose particles is bound in place (all the oscillations are due to the influence of the particles on one another) would have the form

Figure: a row of identical masses connected by identical springs

$$\begin{eqnarray*} A & = & \frac{k}{m} \left[ \begin{array}{rrrrrrr} -2 & 1 & ... ...1 & . & . \\ . & . & . & . & . & . & . \end{array} \right]. \end{eqnarray*}$$