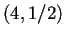 Regla
Regla 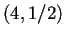 Regla
Regla
Ahora nuestro caso de estudio es un auómata de: 4 estados, radio de vecindad ![]() , regla de evolución
, regla de evolución ![]() y la regla inversa
y la regla inversa ![]() . Para este caso los índices de Welch tienen valores
. Para este caso los índices de Welch tienen valores ![]() y
y ![]() tanto para la regla
tanto para la regla ![]() como para la regla
como para la regla ![]() ; que a continuación se muestran.
; que a continuación se muestran.
|
Los conjuntos ![]() y
y ![]() se presentan a continuación y como se ha dicho anteriormente, se obtuvieron de la misma forma que en el primer ejemplo.
se presentan a continuación y como se ha dicho anteriormente, se obtuvieron de la misma forma que en el primer ejemplo.
En ambos casos se cumple que
![]() y
y
![]() . Tomemos los conjuntos
. Tomemos los conjuntos
![]() y
y
![]() , y especifiquemos los siguientes mapeos
, y especifiquemos los siguientes mapeos ![]() y
y ![]() .
.
Obtengamos las permutaciones ![]() y
y ![]() .
.
|
Con una configuración aleatoria inicial, obtengamos ![]() evoluciones de este autómata y tratemos de reproducir el mismo resultado aplicando las permutaciones en bloque.
evoluciones de este autómata y tratemos de reproducir el mismo resultado aplicando las permutaciones en bloque.
Como en el ejemplo anterior, se obtuvo la misma evolución por las dos formas; observemos que al aplicar las permutaciones del instante ![]() al instante
al instante ![]() , la permutación
, la permutación ![]() se efectuó en las mismas posiciones en que lo había hecho la permutación
se efectuó en las mismas posiciones en que lo había hecho la permutación ![]() del instante
del instante ![]() a
a ![]() ; esto se hizo para hacer más claro el diagrama pero no obedece a alguna condición necesaria, la permutación
; esto se hizo para hacer más claro el diagrama pero no obedece a alguna condición necesaria, la permutación ![]() se puede empezar a aplicar en donde se quiera y el proceso funcionará igual siempre y cuando
se puede empezar a aplicar en donde se quiera y el proceso funcionará igual siempre y cuando ![]() se empiece a aplicar con un corrimiento igual a uno con respecto a
se empiece a aplicar con un corrimiento igual a uno con respecto a ![]() , lo que es similar a un corrimiento de tamaño
, lo que es similar a un corrimiento de tamaño ![]() entre configuraciones.
entre configuraciones.